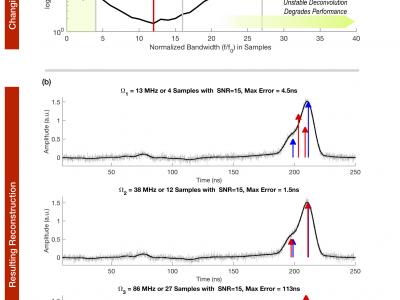
Super-resolution is the art of recovering spikes from their low-pass projections. Over the last decade specifically, several significant advancements linked with mathematical guarantees and recovery algorithms have been made. Most super-resolution algorithms rely on a two-step procedure: deconvolution followed by high-resolution frequency estimation. However, for this to work, exact bandwidth of low-pass filter must be known; an assumption that is central to the mathematical model of super-resolution. On the flip side, when it comes to practice, smoothness rather than bandlimitedness is a much more applicable property. Since smooth pulses decay quickly, one may still capitalize on the existing super-resolution algorithms provided that the essential bandwidth is known. This problem has not been discussed in literature and is the theme of our work. In this paper, we start with an experiment to show that super-resolution in the presence of noise is sensitive to bandwidth selection. This raises the question of how to select the optimal bandwidth. To this end, we propose a bandwidth selection criterion which works by minimizing a proxy of estimation error that is dependent of bandwidth. Our criterion is easy to compute, and gives reasonable results for experimentally acquired data, thus opening interesting avenues for further investigation, for instance the relationship to Cramér-Rao bounds.