Documents
Presentation Slides
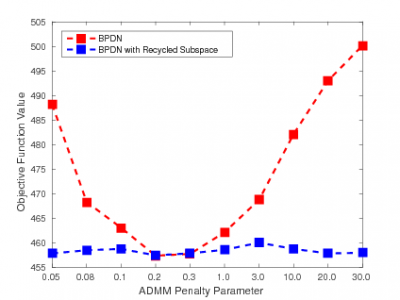
ADMM Penalty Parameter Selection with Krylov Subspace Recycling Technique for Sparse Coding
- Citation Author(s):
- Submitted by:
- Brendt Wohlberg
- Last updated:
- 3 October 2017 - 6:45pm
- Document Type:
- Presentation Slides
- Document Year:
- 2017
- Event:
- Presenters:
- Brendt Wohlberg
- Paper Code:
- WQ-L2.4
- Categories:
- Keywords:
- Log in to post comments
The alternating direction method of multipliers (ADMM) has been widely used for a very wide variety of imaging inverse problems. One of the disadvantages of this method, however, is the need to select an algorithm parameter, the penalty parameter, that has a significant effect on the rate of convergence of the algorithm. Although a number of heuristic methods have been proposed, as yet there is no general theory providing a good choice of this parameter for all problems. One obvious approach would be to try a number of different parameters at each iteration, proceeding further with the one that delivers the best reduction in functional value, but this would involve a substantial increase in computational cost. We show that, when the forward operator of the inverse problem is represented via a transform operator, requiring iterative methods to solve the main linear system arising in the ADMM solution, it is possible to explore a large range of parameters at marginal additional cost, thus greatly improving the robustness of the method to the choice of penalty parameter.

