- Read more about Decreasing the Measurement Time of Blood Sugar Tests using Particle Filtering
- Log in to post comments
- Categories:
 7 Views
7 Views- Read more about Regression, the Periodogram, and the Lomb-Scargle Periodogram
- Log in to post comments
In \cite{Lomb}, Lomb developed a nonlinear regression approach to estimating the frequency of a noisy sinusoid when the measurement times were not equispaced, and a method for correcting the times so that the resulting regression sum of squares appeared very similar to the usual periodogram.
- Categories:
 19 Views
19 Views- Read more about Rate Analysis For Detection Of Sparse Mixtures
- Log in to post comments
Poster for ICASSP 2016
- Categories:
 5 Views
5 Views- Read more about Robust Inference for State-Space Models with Skewed Measurement Noise
- Log in to post comments
Filtering and smoothing algorithms for linear discrete-time state-space models with skewed and heavy-tailed measurement noise are presented. The algorithms use a variational Bayes approximation of the posterior distribution of models that have normal prior and skew-t-distributed measurement noise.
- Categories:
 21 Views
21 Views- Read more about ON PARAMETER ESTIMATION OF SYMMETRIC ALPHA-STABLE DISTRIBUTION
- Log in to post comments
- Categories:
 20 Views
20 Views- Read more about Choosing the diagonal loading factor for linear signal estimation using cross validation
- Log in to post comments
Linear signal estimation based on sample covariance matrices (SCMs) can perform poorly if the training data are limited and the SCMs are ill-conditioned. Diagonal loading (DL) may be used to improve robustness in the face of limited training data. This paper introduces two leave-one-out cross-validation schemes for choosing the DL factor. One scheme repeatedly splits the training data with respect to time, while the other repeatedly splits the out-of-training data with respect to space.
- Categories:
 21 Views
21 Views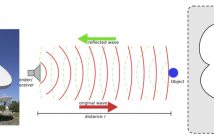
- Categories:
 14 Views
14 Views- Read more about ON PARAMETER ESTIMATION OF SYMMETRIC ALPHA-STABLE DISTRIBUTION
- Log in to post comments
- Categories:
 17 Views
17 Views- Read more about Blind Deconvolution of Sparse But Filtered Pulses With Linear State Space Models
- Log in to post comments
- Categories:
 10 Views
10 Views