
Several distributed real-time signal sensing/monitoring systems require quantization for efficient signal representation. These distributed sensors often have computational and energy limitations. Motivated by this concern, we propose a novel quantization scheme called Approximate Lloyd-Max (ALM) that is nearly-optimal. Assuming a continuous and finite support probability distribution of the source, we show that our ALM quantizer converges to the classical Lloyd-Max quantizer with increasing bitrate.
- Categories:
 149 Views
149 Views
- Read more about Poster for SUPER-RESOLUTION RESULTS FOR A 1D INVERSE SCATTERING PROBLEM
- Log in to post comments
In this work we consider the one-dimensional (1D) inverse scattering problem of super-resolving the location of discrete point scatters satisfying the 1D Helmholtz equation. This inverse problem has important applications in the detection of shunt faults in electrical transmission lines and leaks in water pipelines where usually only low frequency spectral information is available from measurements. We formulate the inverse scattering problem as a sparse reconstruction problem and apply convex optimization to super-resolve the location of point scatters.
- Categories:
 43 Views
43 Views
- Read more about VECTOR APPROXIMATE MESSAGE PASSING FOR QUANTIZED COMPRESSED SENSING
- Log in to post comments
In recent years approximate message passing algorithms have gained a lot of attention and different versions have been proposed for coping with various system models. This paper focuses on vector approximate message passing (VAMP) for generalized linear models. While this algorithm is originally derived from a message passing point of view, we will review it from an estimation theory perspective and afterwards adapt it for a quantized compressed sensing application. Finally, numerical results are presented to evaluate the performance of the algorithm.
- Categories:
 40 Views
40 Views
- Read more about Sketching Discrete Valued Sparse Matrices
- Log in to post comments
The problem of recovering a sparse matrix X from its sketchAXB T is referred to as the matrix sketching problem. Typically, the sketch is a lower dimensional matrix compared to X, and the sketching matrices A and B are known. Matrix sketching algorithms have been developed in the past to recover matrices from a continuous valued vectorspace (e.g., R^N×N ). However, employing such algorithms to recover discrete valued matrices may not be optimal. In this paper, we propose two novel algorithms that can efficiently recover a discrete valued sparse matrix from its sketch.
slides.pdf
- Categories:
 35 Views
35 Views
- Read more about An Interior Point Method for Nonnegative Sparse Signal Reconstruction
- Log in to post comments
We present a primal-dual interior point method (IPM) with a novel preconditioner to solve the ℓ1-norm regularized least square problem for nonnegative sparse signal reconstruction. IPM is a second-order method that uses both gradient and Hessian information to compute effective search directions and achieve super-linear convergence rates. It therefore requires many fewer iterations than first-order methods such as iterative shrinkage/thresholding algorithms (ISTA) that only achieve sub-linear convergence rates.
- Categories:
 11 Views
11 Views
- Read more about ITERATIVE OPTIMIZATION OF QUARTER SAMPLING MASKS FOR NON-REGULAR SAMPLING SENSORS
- Log in to post comments
Non-regular sampling can reduce aliasing at the expense of noise.
Recently, it has been shown that non-regular sampling can be carried
out using a conventional regular imaging sensor when the surface of
its individual pixels is partially covered. This technique is called
quarter sampling (also 1/4 sampling), since only one quarter of each
pixel is sensitive to light. For this purpose, the choice of a proper
sampling mask is crucial to achieve a high reconstruction quality. In
the scope of this work, we present an iterative algorithm to improve
- Categories:
 53 Views
53 Views- Read more about Multilevel Illumination Coding for Fourier Transform Interferometry in Fluorescence Spectroscopy
- Log in to post comments
Fourier Transform Interferometry (FTI) is an interferometric procedure for acquiring HyperSpectral (HS) data. Recently, it has been observed that the light source highlighting a (biologic) sample can be coded before the FTI acquisition in a procedure called Coded Illumination-FTI (CI-FTI). This turns HS data reconstruction into a Compressive Sensing (CS) problem regularized by the sparsity of the HS data. CI-FTI combines the high spectral resolution of FTI with the advantages of reduced-light-exposure imaging in biology.
- Categories:
 9 Views
9 Views
- Read more about Super-Resolution Pulse-Doppler Radar Sensing via One-Bit Sampling
- Log in to post comments
This paper investigates the delay-Doppler estimation problem of a pulse-Doppler radar which samples and quantizes the noisy echo signals to one-bit measurements.By applying a multichannel one-bit sampling scheme, we formulate the delay-Doppler estimation as a structured low-rank matrix recovery problem.Then the one-bit atomic norm soft-thresholding method is proposed to recover the low-rank matrix, in which a surrogate matrix is properly designed to evaluate the proximity of the recovered data to the sampled one.With the recovered low-rank matrix, the delays and Doppler frequencies can be d
- Categories:
 64 Views
64 Views
- Read more about Non-negative Super-resolution is Stable
- Log in to post comments
We consider the problem of localizing point sources on an interval from possibly noisy measurements. In the absence of noise, we show that measurements from Chebyshev sys- tems are an injective map for non-negative sparse measures, and therefore non-negativity is sufficient to ensure unique- ness for sparse measures. Moreover, we characterize non- negative solutions from inexact measurements and show that any non-negative solution consistent with the measurements is proportionally close to the solution of the system with ex- act measurements.
poster.pdf
- Categories:
 12 Views
12 Views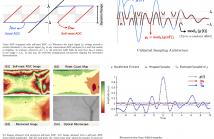
- Read more about Unlimited Sampling of Sparse Signals
- Log in to post comments
In a recent paper [1], we introduced the concept of “Unlimited Sampling”. This unique approach circumvents the clipping or saturation problem in conventional analog-to-digital converters (ADCs) by considering a radically different ADC architecture which resets the input voltage before saturation. Such ADCs, also known as Self-Reset ADCs (SR-ADCs), allow for sensing modulo samples.
- Categories:
 170 Views
170 Views